Equity and Efficiency

A big issue in economics is the trade off between efficiency and equity.

• Efficiency is concerned with the optimal production and allocation of resources given existing factors of production.
• Equity is concerned with how resources are distributed throughout society.

1. Vertical equity is concerned with the relative income and welfare of the whole population e.g. Relative poverty when people have less than 50% of average income. Vertical equity is concerned with how fairly resources are distributed and may imply higher tax rates for high income earners.
2. Horizontal equity is treating everyone in same situation the same. e.g. everyone earning £15,000 should pay same tax rates.

Concepts of efficiency may imply a lack of equity.
For example, the Community Charge (Poll tax) was considered to be economically efficient because a poll tax doesn’t distort economic behavior. (e.g. doesn’t reduce incentives to work). However, by making a millionaire pay the same tax as a poor pensioner, it was considered to be unfair.

A tax on cigarettes can be said to increase social efficiency. The tax makes people pay the social cost of smoking. However, a cigarette tax is also highly regressive. It takes a bigger % of income from low income earners.
Pareto efficiency is concerned with creating a situation where we cannot make one party better off without making another party worse off.
For example, a country may devote 60% of GDP to the manufacture of armaments. In doing this, they may achieve technical and productive efficiency and produce on their production possibility frontier. Therefore from this perspective they are efficient. But, such an economy may have a great deal of inequality, with large portions of the population struggling to have enough to eat.

Bank Bailouts and Equity

From one perspective we may say bailing out banks is an economic necessity as it prevents a collapse in confidence in the banking system. By bailing out banks, we enable a more productively efficient economy. However, from another perspective it seems unfair that the government enables bankers to retain high paying jobs whilst they implement cuts for workers on lower income.

Increased Inequality and Increased Growth

Sometimes, economic policies create a situation where everyone becomes better off (rising real incomes across population). However, those on high incomes gain a bigger % rise in real incomes. The result is that everyone becomes better off, but, there is also greater income inequality. Therefore, some people may feel that relatively they appear worse off compared to others in society.
This is a pareto improvement in economic welfare but also an increase in inequality.
The final point is that there doesn’t have to be a trade off between equality and efficiency. An improvement in efficiency, should generally make the economy better off. There is no reason why improved efficiency has to lead to inequality. It is compatible to improve both efficiency and equity within society.

Factor Markets and Derived Demand

The four factors of production – labor, capital, land and entrepreneurship – are bought and sold in the factor markets. In these markets we reverse the buyer and seller pattern seen in the goods markets; in goods markets firms sell and households buy, but in factor markets firms buy and households sell.

1. Labor: the effort: workers put into producing goods and services such as constructing a house, building a car on an assembly line, designing a new computer, researching a new drug. Laborers are paid wages.

2. Capital: technically, capital refers to the facilities, equipment, inventories and other physical resources to produce goods and service. In the interest of clarity we will call this physical capital to distinguish it from financial capital – the funds necessary to start or maintain the business. Financial capital must be available before a firm can acquire its physical capital. Providers of financial capital are paid interest.

3. Land: Land is a shorthand term that stands for plots of ground and the natural resources contained within. Land can be used to provide housing, agricultural production or raise livestock, just to name a few uses. Land is paid economic rent.

4. Entrepreneurship: Entrepreneurship is the ability to see economic opportunities and organize the other three factors to exploit that opportunity. They are paid profit.

Households own or control these factors and sell them to the producers. They provide the labor; their savings flows into the financial markets and finances physical capital; they own the land; they are the entrepreneurs. Businesses buy the factors from the households and use the inputs to produce goods and services which they then sell to the households. The expenditures of the households are financed by the income they earned selling the factors of production. This makes a giant rotating circle of income and spending. We refer to the interaction between buyers and sellers of inputs as the factor markets.

The factor markets are driven by supply and demand just as any other market. However, there are some differences in the motivation of the sellers and buyers in the factor markets from those in the goods markets, the most obvious of which is the motivation of buyers. In a goods market the buyers want the good based on utility – the benefit consumers enjoy from having and using a good. Buyers of the four factors of production do not gain benefit from having a factor of production; it is a means to an end not the end itself. Buyers of factors of production gain a benefit only if the factor of production adds to their profit; we therefore say that the demand for a factor of production is a derived demand – it is determined by or derived from consumer demand for the final good.

Firms will weigh the cost versus benefit of acquiring each unit of a factor of production; they will expand use of a factor only so long as it is profitable to do so. As with the other economic decisions we have examined, the analysis is conducted at the margin.

Monopoly and Oligopoly

Monopoly

Definition: A market structure characterized by a single seller, selling a unique product in the market. In a monopoly market, the seller faces no competition, as he is the sole seller of goods with no close substitute.

Description: In a monopoly market, factors like government license, ownership of resources, copyright and patent and high starting cost make an entity a single seller of goods. All these factors restrict the entry of other sellers in the market. Monopolies also possess some information that is not known to other sellers.

Characteristics associated with a monopoly market make the single seller the market controller as well as the price maker. He enjoys the power of setting the price for his goods.


Oligopoly

An oligopoly is a market structure in which a few firms dominate. When a market is shared between a few firms, it is said to be highly concentrated. Although only a few firms dominate, it is possible that many small firms may also operate in the market. For example, major airlines like British Airways (BA) and Air France operate their routes with only a few close competitors, but there are also many small airlines catering for the holidaymaker or offering specialist services.

Concentration ratios

Oligopolies may be identified using concentration ratios, which measure the proportion of total market share controlled by a given number of firms. When there is a high concentration ratio in an industry, economists tend to identify the industry as an oligopoly.

Example of a hypothetical concentration ratio

The following are the annual sales, in £m, of the six firms in a hypothetical market:
A = 56
B = 43
C = 22
D = 12
E = 3
F = 1
In this hypothetical case, the 3-firm concentration ratio is 88.3%, that is 121/137 x 100.

Examples

Fixed Broadband services

Fixed broadband supply in the UK is dominated by four main suppliers - BT (with a market share of 32%), Virgin Media (at 20%), Sky (at 22%) and TalkTalk (at 14%), making a four-firm concentration ratio of 86%

Welfare Economics

What is 'Welfare Economics'

Welfare economics is a branch of economics that focuses on the optimal allocation of resources and goods and how this affects social welfare. Welfare economics analyzes the total good or welfare that is achieve at a current state as well as how it is distributed. This relates to the study of income distribution and how it affects the common good.

Welfare economics is a subjective study that may assign units of welfare or utility in order to create models that measure the improvements to individuals based on their personal scales.

Welfare economics uses the perspective and techniques of microeconomics, but they can be aggregated to make macroeconomic conclusions. Because different "optimal" states may exist in an economy in terms of the allocation of resources, welfare economics seeks the state that will create the highest overall level of social welfare.

Some people object to the idea of wealth redistribution because it flies in the face of pure capitalist ideals, but economists suggest that greater states of overall social good might be achieved by redistributing incomes in the economy.

Producer Theory

Just as economists have worked out a theory of consumers they have also developed a theory of producers. This theory explains what is behind the supply functions of markets. While this theory appears to be a general theory explaining how an enterprise behaves, in actuality explaining the behavior of any specific enterprise would be too complex a problem. Some detailed studies of particular firms such as the Ford Motor Company have been written that focus on cultural and organizational aspects of the firm these are not the typical examples of the economic theory of producers. But economics is not concerned with explaining the behavior of any specific firm; instead it is concerned with explaining the behavior of markets. In order to explain the behavior of a typical firm in a market it is not necessary to have a completely realistic and detailed model of firms. All that is required is a model that captures the market-relevant influences of the average and allows the individual differences to average out.

The key concept for a firm is its cost function. The cost function gives the total costs of the firm as a function of its level of production. Let q be the annual rate of output of the firm. Its cost function is total costs C given as as a function of q; i.e., C=f(q). Usually the cost function is represented as C(q).

If the producer can sell the output at a price p then the revenue received is just the price p times the output q. The revenue as a function of q is shown in the above graph as a green line.

The net profit for the firm is the difference between the revenue of pq and the cost C(q).  A producer wants to produce at a level where the profit is the greatest ; i.e., the producer will choose a level of consumption of x such that the profit is a maximum. Note that when the profit is a maximum the slope of the profit function is zero. This means that at the level of q where profit is a maximum the increase in profit from another unit of production is zero. This is equivalent to saying that the increase in revenue from another unit of production is exactly equal to the increase in cost of producing that unit.


The increase in revenue from producing another unit is just the price of the product. The increase in cost can be computed from the cost function. This increase in cost for producing another unit is called the marginal cost of another unit. The marginal cost may decrease with increasing output over some range but beyond some level of production the marginal cost goes up with increasing output.

The marginal cost function is what determines the level of output where profit is a maximum. If the market price of the product is plotted as a horizontal line, as is done in the above graph, then the profit-maximizing output is the output where the price line intersects the marginal cost function. Thus where the price line intersects the marginal cost curve gives the quantity which would be supplied at that price. If this price and quantity data are plotted in a different graph to construct the supply schedule for the firm one finds that the marginal cost curve is just being replotted. In other words,

The supply curve is exactly the same as the marginal cost curve, as least for prices above some minimum. If the price is too low the firm may find that it is most profitable to supply zero units. This would be the case if the price is so low the firm cannot avoid a loss. The upward sloping of the supply curve is just the increasing marginal cost of the increasing production.

There is another cost function that is important for a producer. It is the average cost, the total cost divided by output.

The relationship of the marginal cost curve and the average cost curve is best seen geometrically from the total cost curve. Marginal cost corresponds to the slope of the total cost curve. Average cost corresponds to the slope of a line drawn between a point on the total cost curve and the origin.

When the slope of the tangent to the total cost curve is the same as the slope of the line drawn to the origin then the marginal cost and the average cost are the same. This point is where average cost is a minimum.

There is yet one more cost curve. If total cost is not zero when output is zero that level of cost is called fixed cost. This would be the level of cost in factory that would have to be paid out for maintenance, insurance, security etc.


If fixed costs are subtracted from total costs the difference is called variable costs. Variable costs include the cost of raw materials, labor, power and so forth. If the level of variable costs is divided by output the result is called average variable cost. When marginal cost is equal to average variable cost then average variable cost is at its minimum level.
     

Consumer Theory

The aim of this section is to explain a fundamental problem in economics, the derivation of a consumer’s demand function, in a very simple way. The article is organized as follows:

• Conceptual review of assumptions in demand theory
• Description of the Utility Maximization Problem
• Derivation of the Expenditure Minimization Problem

Assumptions

The consumer theory assumes that the consumer is rational. This implies that his preferences satisfy the following properties: 1. They are complete; that is, given any set of possible bundles of goods, the consumer is always capable of deciding which one is preferable to the others and then ranking them in terms of preference.
2. They are reflexive; it means that any bundle is at least as good as itself.
3. They are transitive; meaning that if a bundle is preferred to a bundle 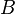, and this bundle is preferable to a third bundle , then it is implied that the first bundle will be preferred to the bundle .
4. They are continuous; there are no big jumps in the ranking of alternatives.
The fulfillment of these properties ensures that consumer’s preferences are consistent and can be represented by an utility function, such that if bundle is preferred to bundle , then
The locus of all bundles that give a certain level of utility to the consumer constitutes an indifference curve (or level curve), which is the usual way of representing preferences. Nevertheless, in spite of these four properties, there is still the possibility of having “special cases” such as the existence of perfect substitutes or perfect complements, among others, which lead to special shapes for the indifference curves. For avoiding these cases, two additional properties are assumed:
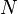5. Preferences are monotonic, or “more is preferred to less”; this implies that, given any set of two bundles, if one of them contains at least as much of all goods and more of one good than the other, then the first bundle will be preferred to the second.
6. Preferences are convex; that is, any combination of two equally preferable bundles will be more desirable than these bundles.
These five properties confer a special shape to level curves: they are downward slopping and convex.

Utility Maximization Problem

This section develops the Utility Maximization Problem (UMP) for the simplest case of only two goods. The model can easily be generalized to goods.
Assume that there are two goods, and , whose prices are and , respectively. The consumer has a fixed amount of income, , for spending on consumption, and his preferences are represented by a generic utility function, , with 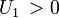, . The consumer’s aim is to obtain the maximum possible utility but he is constrained by his level of income. He cannot spend more than , thus he faces a budget constraint: ¹

Formally, the problem can be formulated as follows:
Max subject to

And it can be solved by the Lagrange Multipliers method:
Max


The first order conditions (FOC) are:




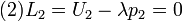
Note that conditions and 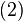imply that . That is, the marginal rate of substitution (MRS) must be equal to the relation of prices, and it means that the indifference curve must be tangent to the budget constraint.

The second order conditions (SOC) are:


It can be demonstrated that the SOC imply that indifference curves are convex. The reciprocal is true only for the case of two goods.
The solutions to the FOC are ,,.They depend on prices and income, thus they can be written as ,,.The functions and ,< are the Marshallian Demand Functions. They represent the amount of goods and , that the consumer is willing to purchase given their prices, income and tastes.
Another concept that emerges from the UMP is the Indirect Utility Function, and it can be obtained by replacing the Marshallian demands into the utility function. By definition, it also is a function of prices and income, then it can be written as . Intuitively, it represents the maximum utility that the consumer can achieve for any given values of .
Note that, because of the Envelop Theorem, it must be the case that . It implies that the Lagrange multiplier can be thought as the marginal utility of income. That is, it represents the rate of change of the maximum utility that is derived from an infinitesimal rise in income.

---

¹ Striclty, the constraint is , but the monotonicity assumption ensures that he will spend all his income.

Expenditure Minimization Problem

The Expenditure Minimization Problem (EPM) is the dual problem of the UMP and it can be thought as follows. Consider a consumer who gets utility through the consumption of the two goods. In this case, there is no restriction on the income to be spent, but the consumer must be on a certain level curve, . Given this constraint, his objective is to reach this indifference curve with the minimum possible expenditure. Therefore, the problem is:

Min subject to

Again, this constrained optimization can be solved by the Lagrange Multipliers method:
Min


The FOC of this program are:





And the SOC are:



Note that conditions and 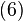imply the same tangency condition than the UMP: . In this program, it means that the expenditure function must be tangent to the indifference curve .
Solving equations to gives the optimal levels of 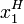,,.The demand functions and are the Hicksian (or Compensated) Demand Functions. Note that these demands depend on prices and the utility level, therefore they are denoted ,,.

The function resulting from replacing the Hicksian demands into the expenditure function gives the minimum expenditure necessary to reach for any given values of . It is called the Indirect Expenditure Function and is denoted .
Again, the Lagrange multiplier has a special interpretation. The Envelop Theorem implies that , meaning that the Lagrange multiplier represents the rate of change of the expenditure function given a change in the utility level to reach.